The magnetic field a distance
 from an infinite current loop carrying current from an infinite current loop carrying current 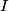 is is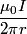 This follows immediately from Ampere's Law. Therefore, the magnetic field at the leftmost wire will be 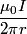 The force on the leftmost wire will therefore be 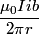 As we found in Problem 54, this force is directed to the left. The magnetic field at the rightmost wire will be 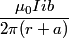 As we found in Problem 54, this force is directed to the right. The forces on the bottom and the top of the wire will cancel, because the magnetic fields are the same, but the currents are in opposite directions. Therefore, the net force on the wire is 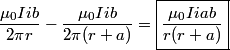 Therefore, answer (D) is correct. |